Designing with op amps for low noise

BY BRIAN BLACK, Product Marketing Manager, 
& GLEN BRISEBOIS, Senior Applications Engineer,
Linear Technology
www.linear.com

The realities of physics prevent any of us from attaining the ideal op amp with perfect precision, zero noise, and infinite open-loop gain, slew rate, and gain-bandwidth product. But we expect successive generations of amplifiers to be better than the previous ones. What, then, should we make of low 1/f noise op amps? 

Back in 1985, George Erdi of Linear Technology designed the LT1028. For over 30 years, it has remained the lowest-voltage-noise op amp available at low frequency with 0.85-nV/√Hz input voltage noise density at 1 kHz and input voltage noise of 35 nV_P-P over 0.1 to 10 Hz. It wasn’t until this year that a new amplifier, the LT6018, challenged the LT1028’s position with 0.1- to 10-Hz input voltage noise of 30 nV_P-P and a 1-Hz 1/f corner frequency, although its wideband noise is 1.2 nV/√Hz. So the LT6018 is the lower-noise choice for lower-frequency applications, while the LT1028 provides better performance for many wideband applications, as shown in Fig. 1 .

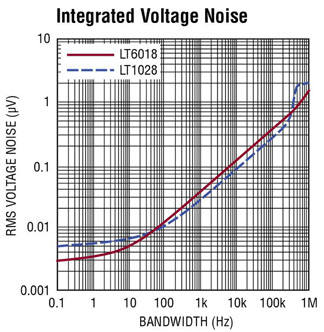
Fig. 1: LT1028- and LT6018-integrated voltage noise.

A noisy noise annoys

But there is more to designing low-noise circuits than choosing the lowest-voltage-noise density (e_n ) amplifier for a given frequency band. As shown in Fig. 2 , other noise sources come into play, with incoherent sources combining as a root sum of squares.

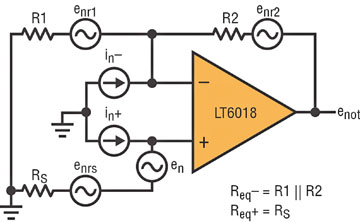
Fig. 2: Op-amp circuit noise sources. 

First, consider resistors as noise sources. Resistors inherently have noise proportional to the square root of the resistance value. At a temperature of 300K, the voltage-noise density of any resistor is e_n = 0.13 √R nV/√Hz. This noise can also be considered as a Norton equivalent current noise: i_n = e_n /R = 0.13/√R nA/√Hz. Resistors, therefore, have a noise power of 17 zeptowatts. Good op amps will have lower noise power than this. For example, the LT6018 noise power (measured at 1 KHz) is only 1 zeptowatt.

In the op-amp circuit of Fig. 2 , the source resistance, gain resistor, and feedback resistor (R_S , R₁ , and R₂ , respectively) all contribute to the circuit noise. When calculating noise, the “per-root-Hertz” used in voltage-noise density can be confusing. But noise power is what adds together, not noise voltage. So to calculate the integrated voltage noise of a resistor or op amp, multiply the voltage-noise density by the square root of the number of Hertz in the frequency band. For example, a 100-Ω resistor has 1.3-μV RMS noise over a 1-MHz bandwidth (0.13 nV/√Ω * √100 Ω * √1,000,000 Hz). For a circuit with a first order rather than brick wall filter, the bandwidth would be multiplied by 1.57 to capture the noise in the higher bandwidth skirt. To express the noise as peak-to-peak rather than RMS, multiply by a factor of 6 (not 2.8, as you would for a sinusoid). With these considerations, the noise of this 100-Ω resistor with a simple 1-MHz low-pass filter is closer to 9.8 μV_P-P .

Also, the op amp has input current noise associated with the current into or out of each input, i_n- and i_n+ . These multiply by the resistances they work into, R₁ in parallel with R₂ in the case of i_n- and R_S in the case of i_n+ , to create voltage noise through the magic of Ohm’s law. Looking inside the amplifier (Fig. 3 ), this current noise is comprised of multiple sources.

Fig. 3: Coherent and incoherent noise sources in an op-amp differential pair.

Considering the wideband noise, each of the two input transistors have shot noise associated with their base, i_ni- and i_ni+ , which are not coherent. The noise from the current source in the input pair tail, i_nt , also creates coherent noise split between the two inputs (i_nt /2β in each). If the resistance seen by the two inputs is equal, the coherent voltage noise at each input is also equal and cancels according to the amplifier’s common-mode rejection capability, leaving primarily the incoherent noise (Fig. 3 ). This is listed as the balanced current noise in datasheets. If the resistance seen at the two inputs is greatly mismatched, then the coherent and incoherent noise components remain and the voltage noise adds as the root sum of squares. This is listed in some datasheets as unbalanced noise current. 

Both the LT1028 and LT6018 have lower voltage noise than a 100-Ω resistor (which, at room temperature, is 1.3 nV/√Hz), so where source resistances are higher, the op amp’s voltage noise will often not be the limiting factor for noise in the circuit. In cases where the source resistances are much lower, the amplifier’s voltage noise will begin to dominate. For very high-source resistances, the amplifier’s current noise dominates, and in the middle, the Johnson noise of the resistors dominates (for well-designed op amps which do not have excessively high noise power). The resistance at which the amplifier current noise and voltage noise are balanced so that neither dominates is equal to the amplifier’s voltage noise divided by its current noise. Since voltage and current noise vary with frequency, so, too, does this midpoint resistance. For an unbalanced source, at 10 Hz, the midpoint of LT6018 is approximately 86 Ω; at 10 kHz, it is about 320 Ω. 

Minimizing circuit noise

So what is the design engineer to do to minimize noise? For processing voltage signals, reducing the equivalent resistance below the amplifier’s midpoint resistance is a good place to start. For many applications, the source resistance is fixed by the preceding stage, often a sensor. The gain and feedback resistors can be chosen to be small. However, because the feedback resistor forms part of the op-amp load, there are limits due to the amplifier’s output drive capability and the acceptable amount of heat and power dissipation. In addition to the resistance seen by the inputs, the frequency should also be considered. The total noise consists of the noise density integrated over the entire frequency. Filtering noise at frequencies higher (and perhaps also lower) than the signal bandwidth is important. 

In transimpedance applications, in which the input to the amplifier is a current, a different strategy is needed. In this case, the Johnson noise of the feedback resistor increases as a square-root factor of its resistance value, but at the same time, the signal gain increase is linear with the resistance value. Hence, the best SNR is achieved with as large a resistance as the voltage capability or the current noise of the op amp allows. For an interesting example, see the back page application on page 26 of the LTC6090 data sheet.

Noise and other headaches

Noise is just one source of error and should be considered within the context of other error sources. Input offset voltage (the voltage mismatch at the op-amp inputs) can be thought of as dc noise. Its impact can be reduced significantly by doing a one-time system calibration, but this offset voltage changes with temperature and time as a result of changes in mechanical stress. It also changes with input level (CMRR) and power supply (PSRR). Real-time system calibration to cancel drift caused by these variables quickly becomes expensive and impractical. For harsh environment applications in which the temperature fluctuates considerably, measurement uncertainty due to offset voltage and drift can dominate over noise. For example, an op amp with 5-μV/°C temperature drift can experience an input-referred shift of 625 μV from –40°C to 85°C due to temperature drift alone. Compared with this, a few hundred nanovolts of noise is inconsequential.

There are many available op amps that have outstanding drift performance. One example is the LT6018 with drift performance of 0.5 μV/°C and a maximum offset spec of 80 μV from –40°C to 85°C. For even better performance, the recently released LTC2057 auto-zero amplifier has a maximum offset voltage of less than 7 μV from –40°C to 125°C. Its wideband noise is 11 nV/√Hz, and its dc to 10-Hz noise is 200 nV_P-P . It is also worth noting that, because of its low input bias current, the LT2057 has much lower current noise than the LT6018. Another benefit of the LTC2057’s low input bias current is that it has very low clock feedthrough compared with many other zero-drift amplifiers. Some of these other zero-drift amplifiers can exhibit large voltage noise spurs when source impedance is high.

In such high-precision circuits, care must also be taken to minimize thermocouple effects, which occur anywhere that there is a junction of dissimilar metals. Even junctions of two copper wires from different manufacturers can generate thermal EMFs of 200 nV/°C — more than 13 times the worst-case drift of the LTC2057. Layout techniques to match or minimize the number of junctions in the amplifier’s input signal path, keeping inputs and matching junctions close together, and avoiding thermal gradients are important in these low-drift circuits.